It is well known that the Electoral College favors small states: every state, no matter how small, gets at least 3 electoral votes, and so small states have more electoral votes per voter. This “well known fact” is, in fact, true.
To state this slightly more formally: if you are a voter in state X, then the probability that your vote is decisive in the Presidential election is equal to the probability that your vote is decisive within your state (that is, the probability that your state would be exactly tied without your vote), multiplied by the probability that your state’s electoral votes are decisive in the Electoral College (so that, if your state flips, it will change the electoral vote winner), if your state were tied.
If your state has N voters and E electoral votes, the probability that your state is tied is approximately proportional to 1/N, and the probability that your state’s electoral votes are necessary is approximately proportional to E. So the probability that your vote is decisive–your “voting power”–is roughly proportional to E/N, that is, the number of electoral votes per voter in your state.
A counterintuitive but wrong idea
The point has sometimes been obscured, unfortunately, by “voting power” calculations that purportedly show that, counterintuitively, voters in large states have more voting power (“One man, 3.312 votes,” in the oft-cited paper of Banzhaf, 1968). This claim of Banzhaf and others is counterintuitive and, in fact, false.
Why is the Banzhaf claim false? The claim is based on the same idea as we noted above: voting power equals the probability that your state is tied, times the probability that your state’s electoral votes are necessary for a national coalition. The hitch is that Banzhaf (and others) computed the probability of your state being tied as being proportional to 1/sqrt(N), where N is the number of voters in the state. This calculation is based (explicitly or implicitly) on a binomial distribution model, and it implies that elections in large states will be much closer (in proportion of the vote) than elections in small states.
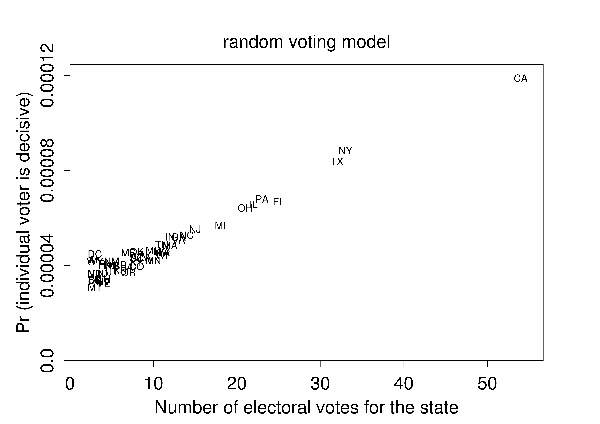
Above is the result of the oversimplified model. In fact, elections in large states are only very slightly closer than elections in small states. As a result, the probability that your state’s election is tied is pretty much proportional to 1/N, not proportional to 1/sqrt(N). And as a result of that, your voting power is generally more in small states than in large states.
Realistically . . .
Realistically, voting power depends on a lot more than state size. The most important factor is the closeness of the state. Votes in so-called “swing states” (Florida, New Mexico, etc.) are more likely to make a difference than in not-so-close states such as New York.
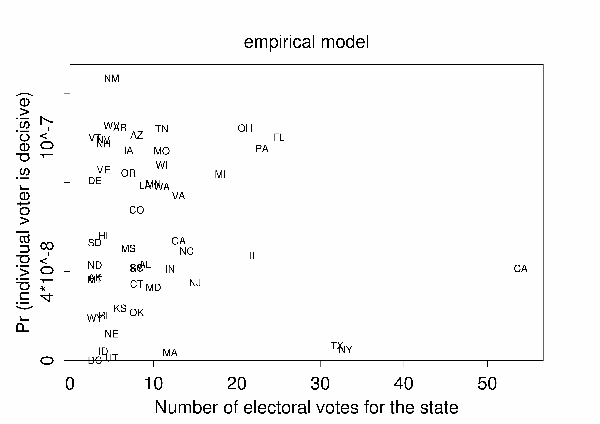
Above is a plot of “voting power” (the probability that your vote is decisive) as a function of state size, based on the 2000 election. These probabilities are based on simulations, taking the 2000 election and adding random state, regional, and national variation to simulate the uncertainty in state-by-state outcomes.

And above is a plot showing voting power vs. state size for a bunch of previous elections. These probabilities are based on a state-by-state forecasting model applied retroactively (that is, for each year, the estimated probability of tie votes, given information available before the election itself).
The punch line: you have more voting power if you live in a swing state, and even more voting power if you live in a small swing state. And, if you’re lucky, your voting power is about 10^(-7), that is, a 1 in 10-million chance of casting a decisive vote.
Actually, though, it’s still rational for you to vote, at least in many of the states.
Boris commented:
Andy,
You start the post off by talking about small and large STATES, but then you talk about VOTERS in small and large states. But aren't these two different things? Electoral systems may benefit BOTH large states and voters in small states simultaneously, it just depends what perspective you take …
Andrew commented:
Boris,
OK, let me be clearer. A voter in New Mexico has higher voting power–that is, has a higher probability of his or her being decisive in the national election–than a voter in California. This is unfair to the Californian in two ways:
First, simple fairness. The New Mexican's vote is more likely to make a difference.
Second, consider the motivations of the candidates. If a certain effort of campaigning is expected to change 1 vote (or 100 or 1000 votes), it would make more sense to put that effort in New Mexico rather than California. If I have less voting power, I am a less appealing target for campaigning and political favors.
The graph above shows that, on average, voting power is higher for voters in small states, so their votes count more. I'd rather have a higher, rather than lower, chance of having my vote matter, and I assume others would feel the same way!
Andrew commented:
Timothy Noah in Slate writes,
The big winners in the Electoral College aren't the small states. They're the large states.
My reply:
This statement is based on a mathematical "voting power" calculation that is in error. As described in the above post (and in detail in our paper), the classical "voting power" measure is appropriate for random voting but not for actual electoral systems