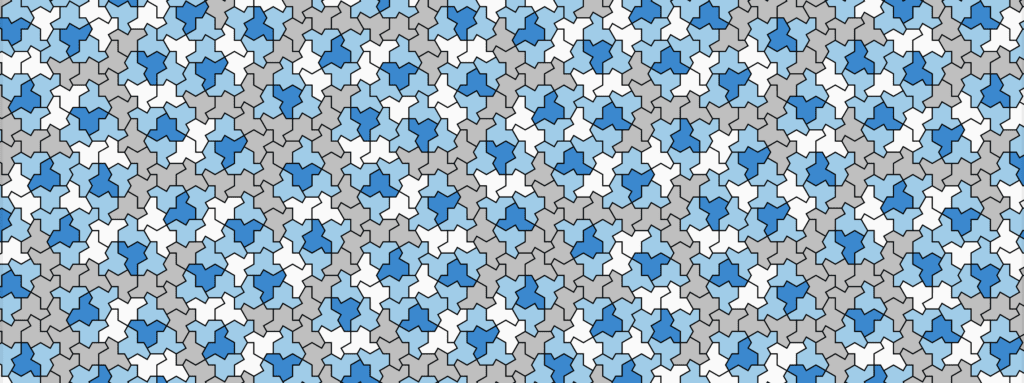
Z in comments points to a new discovery by David Smith, Joseph Samuel Myers, Craig Kaplan, and Chaim Goodman-Strauss, who write:
An aperiodic monotile . . . is a shape that tiles the plane, but never periodically. In this paper we present the first true aperiodic monotile, a shape that forces aperiodicity through geometry alone, with no additional constraints applied via matching conditions. We prove that this shape, a polykite that we call “the hat”, must assemble into tilings based on a substitution system.
All I can say is . . . wow. (That is, assuming the result is correct. I have no reason to think it’s not; I just haven’t tried to check it myself.)
First off, this is just amazing. Even more amazing is that I had no idea that this was even an open problem. I’d seen the Penrose two-shape tiling pattern years ago and loved it so much that I painted a tabletop with it (and send a photo of the table to Penrose himself, who replied with a nice little note, which unfortunately I lost some years ago, or I’d reproduce it here), and it never even occurred to me to ask whether an aperiodic monotile was possible.
This is the biggest news of 2023 so far (again, conditional on the result being correct), and I doubt anything bigger will happen between now and the end of December.
OK, there’s one possibility . . .
Penrose did it with 2 unique tiles, Smith et al. just needed 1, . . . The next frontier in aperiodic tiling is to do it with 0. Whoever gets there will be the real genius.
P.S. Michael in comments points out that the Smith et al. pattern includes mirrored tiles. So let’s call it 1.5. Is there a theorem that you can’t do it with just 1 tile with no mirroring?
Question, as someone whose recent geometry experiences have barely glanced these surfaces.
Can you just pose this as an optimization problem? By my count this shape has 13 vertices, each of which can be represented with 2 parameters, so 26 nominal “parameters”. There’s some further constraint (edges can’t cross, tiles need to fit each other) and flexibility (eg, the tiling occurs independently of rotation, translation, and scaling), but even with, like, rejection sampling of crossed edges, a 26 dimensional space is not that big! Can’t you just explore that space of possible tiles, stochastically shifting the locations of vertices in a manner related to how well the shapes “fit”? With “fitting” the output of either some sort of physics simulation, pieces frictionlessly bouncing around until they click, or by fixing one tile, and doing serial optimization in 3 dimensions (translation in 2D & rotation) to try to place subsequent tiles?
I’d expect it to be a lot more complex than that — just wondering what I’m missing!
Nikolai:
I don’t know, but if you follow the link you’ll see a link to their proof, so you can take a look yourself!
Penrose patented and copyrighted his tiles, which means you can’t buy them to tile your bathroom. Is this new one freely available?
Any patent would have long expired.
But not a copyright. Although there is something weird going on. Either the story isn’t true or there has been an abuse of the patent/copyright system. You can’t patent and copyright the same thing; they’re meant to be complementary designations for different things. And copyrighting/patenting (just one on its own) a mathematical object is already kind of pushing it, like patwnting/cooyrighting a natural object (although I’m aware it happens all the time).
Yes, it is patent free and available for use
There is an argument that Smith et al. use 2 tiles, not 1; they tile they use is sometimes mirrored.
Michael:
Good point. As a wise man once said, you can’t mirror tiles in real life. You can flip them, though, as long as you don’t mind the rough side being face up. So let’s call it 1.5.
I would have thought this was an April Fools item, except it’s not April 1.
The tile appears to be obtained by taking 1 particular Penrose tile, and gluing together 8 copies. Remarkable that it was not found before.
My comment is incorrect. The kites have a slightly different shape.
The note from Penrose was in one of those wooden filing cabinets in our office. I have no idea where the filing cabinets went after we got cubicles.
this will be great for a tile laying boardgame. we need a new shape.
I know I’m late to the game here, but what’s interesting to me is that David Smith is apparently an amateur mathematician. A recent article in the New York Times refers to him as “…a self-described shape hobbyist of Bridlington in East Yorkshire”. Seth Roberts would have loved this. Here’s a link to the NY Times article, minus the paywall: https://archive.is/oLRS9
Alex:
Fun! The news article also quotes Marjorie Senechal, who appeared once on this blog before, in a less happy context.
Regarding the Seth Roberts connection: yes, good point. Smith is an example of Seth’s concept of an insider-outsider: Yes, Smith was an outsider (a hobbyist) but he did have some insider connections with credentialed mathematicians. It seems that both his insider and outsider perspectives were helpful here.
On some visual presentations of this tiling I noticed the tiling occurs among a backdrop of a hexagonal plane whereby the hat fits within hexagons. Interestingly this seems to be a relation of an observed chaos or randomness with a hidden order?
don’t forget Authagraph. they taught me how to tile both a plane and a globe at the same time.
“The hat”: A single shape that can tile the plane aperiodically but not periodically.
Technically the hat isn’t a single shape, there are two hats, one being the mirror of the other.
I think the claim of being a single shape is incorrect.